Genes serve as records of successful solutions that organisms have discovered through trial and error in response to extinction events and processes in the past. Any environmental change could potentially lead to extinction. Considering the successful history of evolution, one might wonder why there isn’t a single organism that encompasses all the best solutions. After all, Homo sapiens is the one species that has made it this far. Why isn’t there a similar phenomenon in nature where only apex organisms outcompete the rest? Similarly, what is the ideal social system? Should we have one political and economic system that incorporates the best practices and lessons learned from human history, or should we have many diverse political systems, including autocratic and tyrannical governments?
These questions relate to a broader inquiry we’ve been asking for centuries: How much bio- or socio-diversity should exist in nature and society, and what are the driving factors behind it? To optimize decision-making for all of humanity, what is the right balance of authority and freedom of choice? What should the appropriate level of taxation be: a large uniform tax typical of socialist societies or low taxes characteristic of capitalist systems? Should insects congregate in swarms or be solitary? Should there be multicellular organisms or single-celled ones? Despite years of exploration, we have yet to arrive at a single solution.
To address these questions, let’s examine the approach of biological and societal structures and information processing systems with the goal of avoiding extinction. For simplicity, let’s assume that extinction events come in two types:
- Environmental changes that cause all organisms without a specific trait to go extinct
- Environmental changes that cause all organisms with a specific trait to go extinct
In this context, I’ll use the term “trait” interchangeably with gene and impact.
A single trait can have both positive and negative effects, providing an advantage in one environment while causing a significant disadvantage in another. For example, an organism’s physical size may provide an advantage in competing for resources and fending off predators, but it was a major disadvantage during the Chicxulub impact event that led to the extinction of large land-based dinosaurs and other large animals about 66 million years ago. Only smaller dinosaurs (e.g., chickens) and smaller animals that could find shelter or burrow underground survived. The question, then, is what the optimal distribution of genes responsible for various traits within species should be and what the ideal number of species should be to maximize survival rates for both types of extinction events.
Type 2 events occur less frequently throughout the history of living organisms but are very abrupt. Examples include mega-volcano eruptions, asteroid impacts, and explosions in the number of species that change the environment. In the past 500 million years, there have been about five major extinction events that resulted in more than 50% of life disappearing, meaning type 2 events happen roughly every 100 million years. The last such event, the Chicxulub impact, occurred less than 100 million years ago, so we are overdue for the next extinction.
Type 1 events occur more frequently but are gradual in nature and have a smaller magnitude, allowing living organisms to adapt through mutation and natural selection. Natural selection is the process of entire species dying because of type 1 environmental changes. Surviving species continue to mutate until they find a solution that halts extinction, and this solution is coded into DNA as a gene that makes the species fit for the environment.
The optimal decision for centralization versus agency is to maximize the utility value according to the formula, which maximizes the number of contributing impacts. In this context, we use our formula as the probability of survival of an expanded definition of life, where the survival of a single trait constitutes the survival of “life.” Traits are divided into two parts: common and specific. Common traits are traits that are grouped together and shared with other species, forming larger groups of “genus” and “family” (Taxonomy (Biology), n.d.) and specific traits are the ones that are not shared with any other species. Those could be new mutations or unique traits for the particular species. Each trait, represented by index i or j, can be a potential solution to the future extinction events:
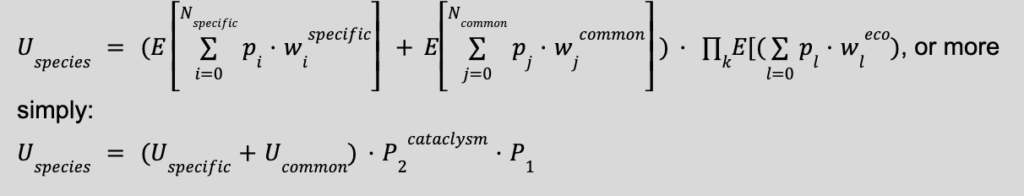
With the type 1 and 2 extinction events the ecological part of equation, wleco, has values:
- w_eco=0, for all groups and species with certain gene missing from the common part, or when l ∉ {j | common set}, which corresponds to slow environment change
- w_eco=0, for all groups and species with certain gene belongs to the common part, or when l ⊂ {j | common set}, which corresponds to abrupt environment change, i.e. extinction event
Let us consider what happens after cataclysm, or the order of occurrence is type 2 followed by type 1 events. This is what would happen immediately after a cataclysmic event, where a few species that survived the extinction will still need to survive through regular environment changes right after.
Let us assume that there is a limited set of all the possible genes that correspond to all the possible traits, where M is the total number of different genes in the set. Let us also assume that in that set there is at least one trait that solves each and every type 1 environment changes.
Consider two extreme configurations:
- In one, we will have one mega-successful species that collected all of the successful traits to survive type 1 environment changes.
- In another, we will have billions of most simple organisms each containing just one trait.
Let us consider what would happen to those configurations after a type 2 cataclysmic event happens followed by a series of type 1 events. The survival probability for that scenario can be written as:
U(species) · P² · ∏(P¹).
Right after type 2 event, the A) species will not survive type 2, as type 2 will target a random trait i, and species A will have all the traits, as a result type 2 will target species A) everywhere, P2 will be zero and the species A) will perish as a result. The whole life will also cease to exist because in that scenario life only comprised A) species. This is why there is such biodiversity on Earth, in the early days of evolution the extinction events of massive magnitude were happening more frequently due to formation of Earth and life was not as diverse or in such large numbers, so magnitude did not have to be large to wipe out species.
On the opposite extreme, right after type 2 event, only one species with an unfortunate trait will perish and the rest will survive. However, right after that event, most of the species will not survive the next, and more gradual environmental change, as they have a very short portfolio of traits. Type 1 events will wipe out all but one species and the next type 1 event will wipe out the remaining species. This is why we do not see super simple organisms that frequently, with the exception of viruses. Even viruses survive only because other, more complex organisms exist and at the cost of having very high mutation rates and losing cellular structure.
Intuitively a solution should be somewhere in the middle between A) and B), but on a more careful consideration we could always find a suboptimal configuration between A and B that would lead to total extinction. For instance, if the common part is missing a lot of genes, the subsequent type 1 events could target traits that were missing from the common part, leading to all the species being wiped out.
The best solution is to have complex organisms with the common part that contains all the genes but one that will be specific to that species:
Σ(a≠o) { pª · wª+pº · wº} . In this configuration there will be as many species as there are different genes. Let us drop for the moment capacity considerations, if the planet can support as many different species.
Let’s consider how the occurrence of type 2 events followed by a series of type 1 events will affect this new configuration. Type 2 will wipe out all the species but the one that is missing the targeted trait. This surviving species will survive subsequent type 1 events with the probability of (M-1/)M, where M is the total number of genes. The higher the number of genes the more the probability approaches 1. This is the best we can do.
This could explain the biodiversity that exists on Earth. A diverse range of species helps to ensure that ecosystems can withstand disturbances such as climate change, invasive species, and natural disasters. It allows for greater adaptability to changing environmental conditions. But most importantly it assures the survival of all of life on Earth.
In societal structures, there are also type 2 and type 1 changes in the ecological and geopolitical environments. Overpopulation, world wars and natural cataclysms happen rarely and abruptly and political, economic changes and regional wars happen steadily throughout human history.
Societal diversity provides the same benefits to protect against massive political or economical upheavals.
Our solution to type 2 and type 1 brings out an unexpected conclusion to social structures: the most stable systems are not having one successful world government, but as many governments as there are political structures. Having democracy as a world system may not be beneficial for human society stability and survival. The fact that the number of types of governments in the modern days can be counted on both hands is concerning. The good news is that it increased from mostly feudal monarchies to several different types: monarchy, theocracy, dictatorships, socialism and capitalism democracy.
Diverse social structures are the key to mankind’s wellbeing, but those structures need to be carefully balanced in order to maximize the odds of our civilization’s survival.

